^ — power function

1. Definition

Power function is the function of kind

x^a

2. Graph

Power function domain depends on power a, but it is always defined for positive real half-axis (0, +∞). Parabola graph for a = 2 is depicted below — fig. 1.

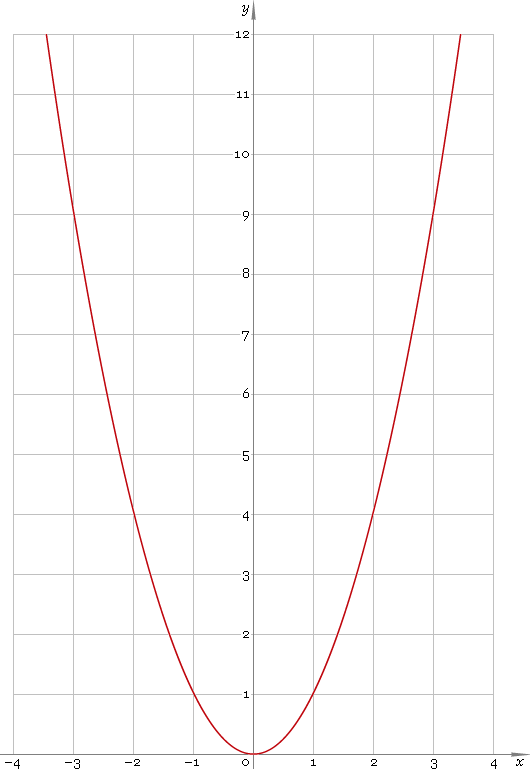Fig. 1. Graph of the power function y = x².

Function codomain as well depends on power a, but it always includes positive half of the real axis (0, +∞).

3. Identities

Negative power:

x^−a = 1 /x^a

Power sum and difference:

x^{a + b} = x^a x^b
x^{a − b} = x^a/x^b

Power product:

x^ab = (x^a)^b

4. Derivative and indefinite integral

Power derivative:

x^a′ = a x^a−1

In particular:

(1 /x)′ = x⁻¹′ = −x⁻² = −1 /x²

Indefinite integral of the power:

∫ x^a dx = x^a+1 / (a + 1) + C

for a ≠ −1, in the case a = −1:

∫ 1 /x dx = ln|x| + C

Here and above C is an arbitrary constant.

5. How to use

To calculate power of the number:

9^2;

To get power of the complex number:

(9+i*7)^(2−i);

To calculate power of the current result:

rslt^2;

To engage the current result as power:

9^rslt;

To get power of the number z in calculator memory:

mem[z]^2;

To engage number z in memory as power:

9^mem[z];

6. Support

Power function of the real argument is supported in free version of the Librow calculator.

Power function of the complex argument is supported in professional version of the Librow calculator.

	Function 1 √ or sqrt
	Function 2 Γ or Gamma
	Function 3 ∏ or Prod
	Function 4 ∑ or Sum
	Function 5 abs
	Function 6 arccos
	Function 7 arccot or arcctg
	Function 8 arccsc or arccosec
	Function 9 arcosh or arch
	Function 10 arcoth or arcth
	Function 11 arcsch
	Function 12 arcsec
	Function 13 arcsin
	Function 14 arctan or arctg
	Function 15 arg
	Function 16 arsech or arsch
	Function 17 arsinh or arsh
	Function 18 artanh or arth
	Function 19 ceil
	Function 20 conj
	Function 21 cos
	Function 22 cosh or ch
	Function 23 cot or ctg
	Function 24 coth or cth
	Function 25 csc or cosec
	Function 26 csch
	Function 27 exp
	Function 28 fact
	Function 29 floor
	Function 30 I
	Function 31 im
	Function 32 J
	Function 33 K
	Function 34 ln
	Function 35 log or lg
	Function 36 ^
	Function 37 rand
	Function 38 re
	Function 39 sec
	Function 40 sech
	Function 41 sign
	Function 42 sin
	Function 43 sinh or sh
	Function 44 tan or tg
	Function 45 tanh or th
	Function 46 Y