| LIBROW |
| Professional | Help | Support | Contacts |
Help 6.27
exp — exponential function
1. Definition
Exponential function is the function of kind
exwhere constant e is selected so that slope of the function at point x = 1 is 45°.
2. Graph
Exponential function is defined everywhere on real axis. Its graph is depicted below — fig. 1.
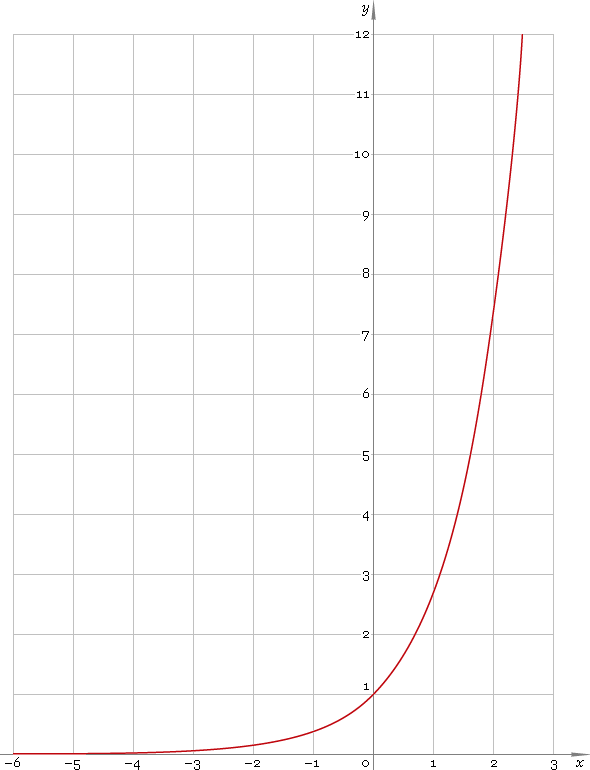 Fig. 1. Graph of the exponential function y = ex.
Fig. 1. Graph of the exponential function y = ex.
Function codomain is positive half of the real axis: (0, +∞).
3. Identities
By definition:
elnx ≡ xNegative argument:
e−x = 1 /exSum and difference of arguments:
ex + y = ex eyex − y = ex/ey
Product of arguments:
exy = (ex)yBase change:
ax = ex lna4. Derivative and indefinite integral
Exponent derivative:
ex′ = exIndefinite integral of the exponent:
∫ ex dx = ex + Cwhere C is an arbitrary constant.
5. How to use
To calculate exponent of the number:
exp(−1);To get exponent of the complex number:
exp(−1+i);To get exponent of the current result:
exp(rslt);To get exponent of the number z in calculator memory:
exp(mem[z]);6. Support
Exponent of the real argument is supported in free version of the Librow calculator.
Exponent of the complex argument is supported in professional version of the Librow calculator.
| Function 1 √ or sqrt |
|
| Function 2 Γ or Gamma |
|
| Function 3 ∏ or Prod |
|
| Function 4 ∑ or Sum |
|
| Function 5 abs |
|
| Function 6 arccos |
|
| Function 7 arccot or arcctg |
|
| Function 8 arccsc or arccosec |
|
| Function 9 arcosh or arch |
|
| Function 10 arcoth or arcth |
|
| Function 11 arcsch |
|
| Function 12 arcsec |
|
| Function 13 arcsin |
|
| Function 14 arctan or arctg |
|
| Function 15 arg |
|
| Function 16 arsech or arsch |
|
| Function 17 arsinh or arsh |
|
| Function 18 artanh or arth |
|
| Function 19 ceil |
|
| Function 20 conj |
|
| Function 21 cos |
|
| Function 22 cosh or ch |
|
| Function 23 cot or ctg |
|
| Function 24 coth or cth |
|
| Function 25 csc or cosec |
|
| Function 26 csch |
|
| Function 27 exp |
|
| Function 28 fact |
|
| Function 29 floor |
|
| Function 30 I |
|
| Function 31 im |
|
| Function 32 J |
|
| Function 33 K |
|
| Function 34 ln |
|
| Function 35 log or lg |
|
| Function 36 ^ |
|
| Function 37 rand |
|
| Function 38 re |
|
| Function 39 sec |
|
| Function 40 sech |
|
| Function 41 sign |
|
| Function 42 sin |
|
| Function 43 sinh or sh |
|
| Function 44 tan or tg |
|
| Function 45 tanh or th |
|
| Function 46 Y |
|
|||||||||||||
|
|||||||||||||